For those of us with no real navigation experience, encountering longitude and latitude coordinates is usually the result of some hiccup using Google Maps. 40.722272, -73.994194? What? Where the heck is that? Why are there so many digits after the decimal?
We’re here to help. And because one degree of longitude and latitude can actually represent very different distances, we might as well start with the very, very basics.
Latitude specifies the north-south position. The equator is 0°, the North Pole 90°, and the South Pole -90°. Latitude lines drawn on a globe resemble a stack of circles of difference sizes, with the largest at the equator.
Longitude specifies the earth-west position. For no reason other than an accident of history, the Prime Meridian, which runs through Greenwich, England, is 0°. Longitude goes up to 180° east and 180° west. Every longitude line runs through both the North and South Pole.
Knowing these definitions, you can pretty easily convert differences in longitude and latitude to distances — if you know the the radius of the Earth off the top of your head and can do trigonometry on the fly. But here’s the shortcut for everyone else.
At the equator, one degree of longitude and latitude both cover about 111 kilometres. When we get away from the equator, this starts getting tricky.
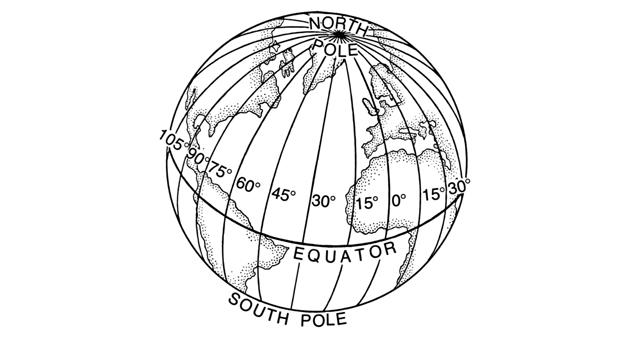
Longitude lines. Pearson Scott Foresman/Creative Commons
As you approach the poles, the distance represented by one degree of difference in longitude gets to zero. This is easy to understand if you envision a globe painted with longitudinal lines, each one a ring passing through the North and South Pole. At these poles — the “ends of the Earth” — the lines are all scrunched up next to each other, and the distance represented by one degree of longitude is effectively zero. Here’s a table summarizing how one degree of longitude changes from the equator to the poles.
But one degree of latitude stays, more the less, the same at 111km. The ever-interesting Jason Kottke has dug up a comment on Stack Exchange, which tells us exactly what the digits in a latitude reading can tell us. It’s a fascinating way to understand the scale of the coordinate system and the things it helps us locate.
The units digit (one decimal degree) gives a position up to 111 kilometers (60 nautical miles, about 69 miles). It can tell us roughly what large state or country we are in.
The first decimal place is worth up to 11.1 km: it can distinguish the position of one large city from a neighbouring large city.
The second decimal place is worth up to 1.1 km: it can separate one village from the next.
The third decimal place is worth up to 110 m: it can identify a large agricultural field or institutional campus.
The fourth decimal place is worth up to 11 m: it can identify a parcel of land. It is comparable to the typical accuracy of an uncorrected GPS unit with no interference.
The fifth decimal place is worth up to 1.1 m: it distinguish trees from each other. Accuracy to this level with commercial GPS units can only be achieved with differential correction.
The sixth decimal place is worth up to 0.11 m: you can use this for laying out structures in detail, for designing landscapes, building roads. It should be more than good enough for tracking movements of glaciers and rivers. This can be achieved by taking painstaking measures with GPS, such as differentially corrected GPS.
Read the whole post to find out what thirteen decimal places can mean. [Stack Exchange]
Top image: Strebe/Creative Commons